The Art of Variable-Speed Pumping to a (Delta-) T
Variable-speed hydronic circulation has been around for years. With the advent of packaged controls on pumps, it is easier than ever to implement.
To take a closer look at the concept of variable-speed pumping, I asked professional engineer and manager of application engineering for Taco Inc. Greg Cunniff and Warwick, R.I.-based plumbing and heating specialist William J. Riley to explain the best applications and key benefits of the technology.
Convection-Heat-Transfer Equation
The speed of a variable-speed pump is adjusted automatically based on heating- or cooling-load demand. To understand how, let's take a quick look at the convection-heat-transfer equation, which, for water, is:
gpm = Btuh ÷ (∆T × 500)
where:
gpm = the flow rate, in gallons per minute, needed to meet heating- or cooling-load demand
Btuh = the heat or cooling, in British thermal units per hour, required for a zone
∆T = the delta-T, or designed temperature drop, across a piping circuit (for heating, design delta-T typically is 20°F; in many radiant-floor-heating and chilled-water-cooling systems, however, it usually is about 10°F)
500 = the specific heat of water, in British thermal units per minute per gallon per hour per Fahrenheit degree (8.33 lb per gallon times 60 min per hour times 1 Btu per pound per Fahrenheit degree)
Sample Project
Consider the example of a small restaurant with a heat gain of 75,000 Btuh and an outdoor design temperature of 95°F. Three zones of fan coils, each with a cooling load of 25,000 Btuh, are needed. Each zone is designed to a 10°F delta-T and has a flow rate of 5 gpm. With this information, the boiler and chiller supply and return pipes, distribution header, and zone piping can be sized.
Pipe-sizing guidelines are based on minimum and maximum flow velocity and maximum head loss. Recommended design parameters are velocities of 2 fps to 8 fps at a head loss of no more than 4 ft per 100 ft. In smaller pipe, head loss of 4 ft per 100 ft rules, resulting in a maximum velocity of 4 fps, above which noise is likely. In larger pipe, a velocity of 8 fps rules, resulting in pressure drops below 4 ft per 100 ft. Larger pipe can withstand higher velocities without noise.
According to Riley, president of William J. Riley Plumbing & Heating Company Inc., determining the piping arrangement is next. He said our example calls for 11/4-in. pipe and 15-gpm flow. He said he would branch into 1-in. lines for each fan-coil zone at the chiller header before doing the same, only in reverse, for the return side of the system.
Next up: estimating the head loss of the piping system. Riley measures the longest zone from the discharge side of the pump all of the way around the system, through the chiller, and back to the suction side of the pump. For this application, the longest run is 150 ft of pipe, including the fan coil.
To allow for additional pressure drop through fittings, Riley said he multiplies the length of the longest run of pipe by 1.5. Returning to our example, 150 ft multiplied by 1.5 equals 225 ft. That total equivalent length, then, is multiplied by 0.04 (representing 4 ft of head loss per 100 ft of straight, properly sized pipe, based on the maximum pressure drop of 4 ft per 100 ft), yielding 9 ft of head loss.
To the head loss of the pipe the pressure drop of the other components in the system is added. The pressure drop of a chiller may be 5 ft, an air separator 1 ft, a fan coil 2 ft, and control and balance valves 10 ft. The pump, then, must be sized to provide 15 gpm while overcoming a head loss of 27 ft (9 + 5 + 1 + 2 + 10).
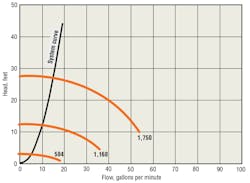
System Curve
With flow a function of head squared, a system curve (parabola) can be plotted through two points on a pump curve. The actual operating point of the system is where the system curve intersects the pump curve. The system in our example requires 15 gpm only when all zones are calling and the outdoor temperature is 90°F.
The building will need less heat when zone valves begin to close, Riley said. If only two zones are calling, demand will drop to 50,000 Btu; if only one zone is calling, demand will drop to 25,000 Btu, meaning flow will be higher than needed. This soon will translate to chiller short-cycling and substantially impact overall system efficiency.
The Perfect Hydronic Storm: Falling Delta-T
Another concern is pressure differential within a system. As zone valves close, a system curve intersects a pump curve at higher and higher pressure differentials. Generally, the greater the pressure differential, the greater the flow velocity, which quickly can lead to noise.
One method of dealing with noise involves installation of a pressure-differential bypass valve, which prevents flow when all zones are calling. As zone valves close, increasing pressure differential, a bypass valve opens, allowing excess pressure and flow to pass through on their way to the suction (inlet) side of a pump.
Another solution for noise involves use of a variable-speed pump.
With only two zones calling, delta-T drops to about 7°F (a 25-percent difference), and with only one zone calling, it drops to about 3°F, a 70-percent decrease.
The dilemma of falling delta-T can be solved using a fixed-delta-T, variable-speed pump, Riley said. With a fixed delta-T, flow varies automatically. So, rather than searching for the point at which a system curve intersects a pump curve, we know the pump curve will self-adjust (Figure 1).
The objective is to satisfy the heat gain of a structure as efficiently as possible. The way to do that is to allow a pump to adjust its speed to deliver the required heat. By maintaining a consistent delta-T, we can vary flow as needed to ensure optimal performance and heat transfer.
DELTA-P VS. DELTA-T
Another pump-control concept concerns pressure differential (delta-P). As a pump changes its speed to maintain a fixed system pressure differential, system delta-T fluctuates, often decreasing.
How does reduced delta-T affect a system? Consider its impact on a modulating condensing boiler. If the system is designed for a delta-T of 20°F, but achieves only 12°F to 15°F, the amount of run time the boiler spends below the point of flue-gas condensation will be affected.
If a reset control is telling a boiler supplying heat to radiators to fire to a high limit of 142°F on a 20°F day, a delta-P circulator programmed based on estimated system head loss may wind up sending 130°F water to the boiler. That is the condensing point, which makes the boiler work at, say, 86 percent annual fuel-utilization efficiency (AFUE).
A pump programmed to deliver a 20°F delta-T, on the other hand, will send 122°F water back to the boiler, creating more condensate, allowing a boiler to operate at an AFUE of 89 percent.
John Vastyan is president of Common Ground (www.seekcg.com), a specialist in marketing communications and business-to-business public relations serving the hydronic, geothermal, radiant-heat, HVAC, and plumbing-and-mechanical industries.